Understanding Trigonometry Formulas: A Comprehensive Guide

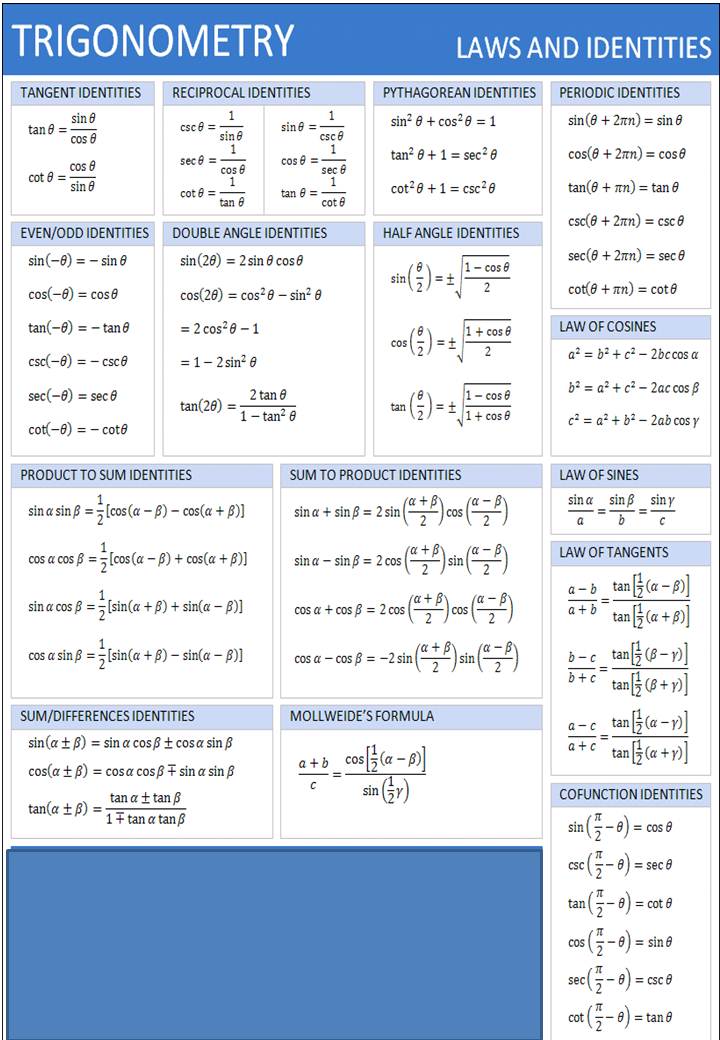
Trigonometry formulas are essential tools in mathematics that help us understand the relationships between the angles and sides of triangles. Whether you are a student trying to grasp the basics or a professional needing to apply these concepts in real-world situations, mastering trigonometric formulas is crucial. In this article, we will explore the various trigonometry formulas, their applications, and how they can be used to solve different types of problems.

In the realm of mathematics, trigonometry plays a vital role, especially in geometry, physics, engineering, and many applied sciences. It revolves around six fundamental functions: sine (sin), cosine (cos), tangent (tan), cosecant (csc), secant (sec), and cotangent (cot), each defined in relation to a right triangle. Understanding these functions is crucial for anyone looking to delve deeper into mathematical studies or applications.

This article aims to provide a thorough understanding of trigonometry formulas, including their derivations, applications, and practical examples. Whether you are preparing for exams, working on a project, or simply looking to enhance your knowledge, this guide will serve as a valuable resource.

Table of Contents

• Trigonometry Basics
• Trigonometric Functions
• Fundamental Trigonometry Formulas
• Trigonometric Identities
• Applications of Trigonometry
• Advanced Trigonometry Formulas
• Examples and Problems
• Conclusion

Trigonometry Basics

Trigonometry, derived from the Greek words "trigonon" (triangle) and "metron" (measure), is the study of the relationships between the angles and sides of triangles, particularly right triangles. The three sides of a right triangle are referred to as the opposite side, adjacent side, and hypotenuse. The hypotenuse is the longest side, opposite the right angle.

In trigonometry, angles are typically measured in degrees or radians. A full circle is 360 degrees or 2π radians. Understanding the basic terminology and relationships in trigonometry is crucial for mastering the formulas that will be discussed later.

Trigonometric Functions

The six primary trigonometric functions are defined based on the ratios of the sides of a right triangle:

• Sine (sin): The ratio of the length of the opposite side to the length of the hypotenuse.
• Cosine (cos): The ratio of the length of the adjacent side to the length of the hypotenuse.
• Tangent (tan): The ratio of the length of the opposite side to the length of the adjacent side.
• Cosecant (csc): The reciprocal of sine (1/sin).
• Secant (sec): The reciprocal of cosine (1/cos).
• Cotangent (cot): The reciprocal of tangent (1/tan).

Fundamental Trigonometry Formulas

Here are some of the fundamental trigonometry formulas that are commonly used:

• Pythagorean Identity: sin²(θ) + cos²(θ) = 1
• Angle Sum Formulas:
  • sin(α + β) = sin(α)cos(β) + cos(α)sin(β)
  • cos(α + β) = cos(α)cos(β) - sin(α)sin(β)
• Angle Difference Formulas:
  • sin(α - β) = sin(α)cos(β) - cos(α)sin(β)
  • cos(α - β) = cos(α)cos(β) + sin(α)sin(β)

Trigonometric Identities

Trigonometric identities are equations that involve trigonometric functions and are true for all values of the variables involved. Some key identities include:

• Reciprocal Identities:
  • csc(θ) = 1/sin(θ)
  • sec(θ) = 1/cos(θ)
  • cot(θ) = 1/tan(θ)
• Co-Function Identities:
  • sin(90° - θ) = cos(θ)
  • cos(90° - θ) = sin(θ)
• Even-Odd Identities:
  • sin(-θ) = -sin(θ)
  • cos(-θ) = cos(θ)

Applications of Trigonometry

Trigonometry has numerous applications across various fields, including:

• Architecture: Designing buildings and structures.
• Engineering: Calculating forces and angles in mechanical systems.
• Physics: Understanding wave motion, oscillations, and circular motion.
• Astronomy: Measuring distances to celestial bodies.

Advanced Trigonometry Formulas

As one delves deeper into trigonometry, several advanced formulas become essential:

• Law of Sines: a/sin(A) = b/sin(B) = c/sin(C)
• Law of Cosines: c² = a² + b² - 2ab*cos(C)

Examples and Problems

To better understand how to apply trigonometry formulas, consider the following examples:

• Example 1: Calculate the height of a tree if the angle of elevation from a point 50 meters away is 30°.
• Example 2: Find the distance between two points on a map using the Law of Cosines.

Conclusion

In summary, understanding trigonometry formulas is essential for anyone engaged in mathematics or its applications. The relationships defined by these formulas provide the foundation for solving complex problems in various fields. We encourage you to practice these formulas and explore their applications further.

Feel free to leave a comment below if you have any questions or insights, and don’t forget to share this article with others who may benefit from it. Additionally, check out our other articles for more in-depth information on related topics.

Thank you for reading, and we look forward to seeing you back on our site for more informative content!